# gst: apropos of absorbing targets, persistence exponents of self-interacting random walks

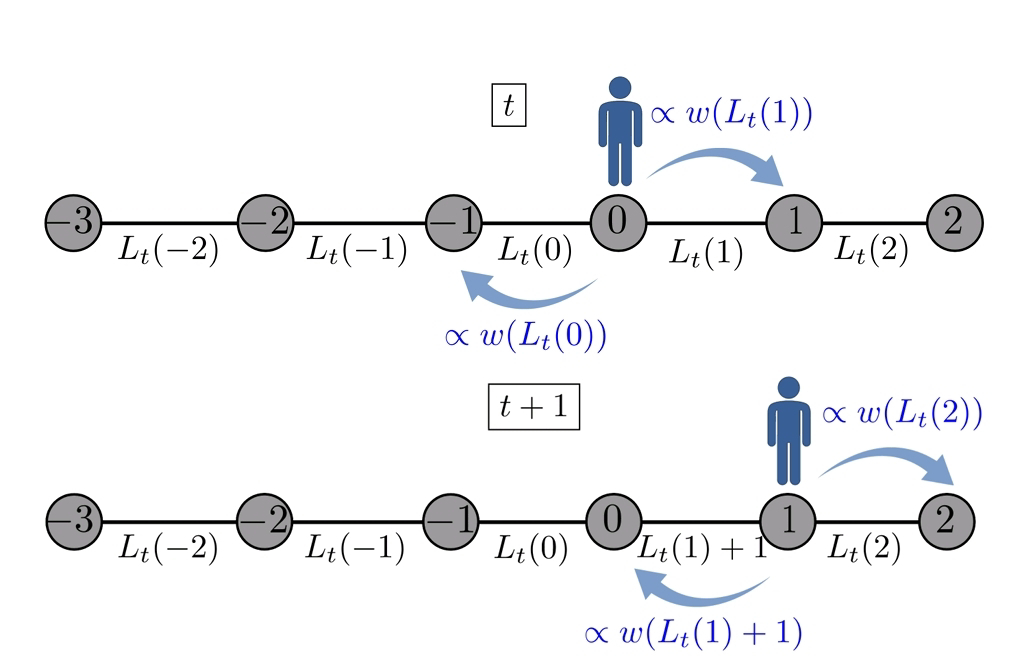
<< The persistence exponent, which characterizes the long-time decay of the survival probability of stochastic processes in the presence of an absorbing target, plays a key role in quantifying the dynamics of fluctuating systems. Determining this exponent for non-Markovian processes is known to be a difficult task, and exact results remain scarce despite sustained efforts. >> 

In their Letter, AA << consider the fundamental class of self-interacting random walks (SIRWs), which display long-range memory effects that result from the interaction of the random walker at time 𝑡 with the territory already visited at earlier times 𝑡′ <𝑡. (AA)  compute exactly the persistence exponent for all physically relevant SIRWs. As a byproduct, (They) also determine the splitting probability of these processes. >>

<< Besides their intrinsic theoretical interest, these results provide a quantitative characterization of the exploration process of SIRWs, which are involved in fields as diverse as foraging theory, cell biology, and nonreversible Monte Carlo methods. >>

J. Brémont, L. Régnier, et al. Persistence Exponents of Self-Interacting Random Walks. Phys. Rev. Lett. 134, 197103. May 16, 2025.

https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.134.197103

arXiv:2410.18699v1 [cond-mat.stat-mech]. 

https://arxiv.org/abs/2410.18699

Also: walk, walking, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, walk, walking, self-interacting random walk, walker self-repulsion, walker self-attraction, stochasticity, absorbing targets.

# gst: toy model of turbulent shear flow using vortons.

AA << introduce a toy model for shear flows, exploiting the spatial intermittency and the scale separation between large-scale flows and small-scale structures. The model is highly sparse, focusing exclusively on the most intense structures, which are represented by vortons—dynamically regularized quasisingularities that experience rapid distortion from the large-scale shear. The vortons, in turn, influence the large-scale flow through the subgrid stress tensor. >>

<< Despite its simplicity, the model displays an interesting transition between two distinct regimes: (i) a laminar regime, where dissipation is entirely attributed to the large-scale flow and the vortons dynamics is essentially diffusive, and (ii) a turbulent regime, in which most of the dissipation arises from the vortons. These regimes correspond to different scalings of dissipation and the Grashof number as functions of the Reynolds number, with power-law relationships that resemble those observed in classical turbulence. >>

Wandrille Ruffenach, Lucas Fery, Bérengère Dubrulle. Toy model of turbulent shear flow using vortons. Phys. Rev. Fluids 10, 054601. May 1, 2025.

https://journals.aps.org/prfluids/abstract/10.1103/PhysRevFluids.10.054601

arXiv:2501.05779v2 [physics.flu-dyn]. 

https://arxiv.org/abs/2501.05779

Also: turbulence, intermittency, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, turbulence, intermittency, transitions, vortons

# life: to protect freedoms, not just funding.

<< At Harvard University in Cambridge, Massachusetts, and Columbia University in New York City, cuts to previously granted federal research funds have been accompanied by demands from the government. If enacted, the changes to departmental oversight, disciplinary procedures, campus police and governance structures would allow the government to dictate hiring, student admissions and disciplinary decisions. >>

<< So far, the US science community has been outspoken about the threats that funding cuts pose to health, innovation and the economy. Yet, few researchers have decried the devastation wreaked on science by attacks on civil liberties and the rule of law. >>

Andrew M. Leifer, Andrea J. Liu, Sidney R. Nagel. US researchers must stand up to protect freedoms, not just funding. Nature 641, 592-593. May 13, 2025.

https://www.nature.com/articles/d41586-025-01466-5

Also: people who turn career into a game playing ... FonT. May 31, 2017.

https://flashontrack.blogspot.com/2017/05/s-game-people-who-turn-career-into-game.html

Also: minimal risk, in FonT 

https://flashontrack.blogspot.com/search?q=minimal+risk

Also: acad, in Font https://flashontrack.blogspot.com/search?q=acad.  acad, in Notes (quasi-stochastic poetry) https://inkpi.blogspot.com/search?q=acad

Also:  dance, game, transition, sars* covid* (aka 1or2achoos), forms of power, Mr. Donald, are you ready, in https://www.inkgmr.net/kwrds.html 

Also: nefst (e.g. not embedded, freestyle thinker) 

https://quod.lib.umich.edu/m/middle-english-dictionary/dictionary/MED20173/track

Keywords: life, acad, game, dance, transitions, nefst, 1or2achoos, minimal risk, turn career, forms of power, Mr. Donald, are you ready.

# gst: accelerated first detection in discrete-time quantum walks using sharp restarts.

<< Restart is a common strategy observed in nature that accelerates first-passage processes, and has been extensively studied using classical random walks. In the quantum regime, restart in continuous-time quantum walks (CTQWs) has been shown to expedite the quantum hitting times [Phys. Rev. Lett. 130, 050802 (2023)]. >>

 Here, AA << study how restarting monitored discrete-time quantum walks (DTQWs) affects the quantum hitting times. (They) show that the restarted DTQWs outperform classical random walks in target searches, benefiting from quantum ballistic propagation, a feature shared with their continuous-time counterparts. >>

Kunal Shukla, Riddhi Chatterjee, C. M. Chandrashekar. Accelerated first detection in discrete-time quantum walks using sharp restarts. Phys. Rev. Research 7, 023069. Apr 21, 2025.

https://journals.aps.org/prresearch/abstract/10.1103/PhysRevResearch.7.023069

Also: walk, random, network, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, networks, randomness, walk, random walk, quantum walk, stochasticity, sharp restart.

# gst: biased random walks on networks with stochastic resetting.

<< This study explores biased random walk dynamics with stochastic resetting on general networks. (AA) show that the combination of biased random walks and stochastic resetting makes significant contributions by analyzing the search efficiency. (They) derive two analytical expressions for the stationary distribution and the mean first passage time, which are related to the spectral representation of the probability transition matrix of a biased random walk without resetting. These expressions can be used to determine the capacity of a random walker to reach the specific target and probe a finite network. >>

AA << apply the analytical results to two types of networks, pseudofractal scale-free webs and T-fractals, which are constructed through an iterative process. (They) also extend a strategy to explore other complex structure networks or larger networks by leveraging the spectral properties. >>

Anlin Li, Xiaohan Sun. Biased random walks on networks with stochastic resetting. Phys. Rev. E 111, 054309. May 16, 2025.

https://journals.aps.org/pre/abstract/10.1103/PhysRevE.111.054309

Also: walk, random, network, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, networks, randomness, random walk, stochasticity, stochastic resetting.

# life: the pervasive "soft power" of ancient India.

<< One of the big contentions of popular historian William Dalrymple’s latest book “The Golden Road: How Ancient India Transformed the World,” which came out in the United States a few weeks ago, is that the Indian subcontinent’s connections to the West, especially via the Roman Empire, were far richer than those of China (i.e. the “Silk Road” cited). Once the might of Rome reached Egypt and the maritime routes of the Red Sea, it brought the customers of the Mediterranean to India’s doorstep. It also saw Indian philosophy and mathematics travel west and east. >>

<< Once their economic links to the West thinned with the collapse of the Roman Empire, South Indian merchant guilds turned east, embarking on trade and contacts that spread Indian religion and ideas across a wide expanse of Asia and underlay the grandeur of centuries-old temple complexes like Angkor Wat in Cambodia or Borubudur in Indonesia. >>

<< it’s one of the great soft power miracles of world history, because unlike Islam and unlike quite a lot of Christianity, no one took Buddhism at the point of a sword. No one imposed Buddhism at any point. It was the sophistication of its ideas and particularly its attractiveness to the merchant classes, bizarrely. The Buddhist monasteries act as banks, as factories and as caravanserais. >>

Ishaan Tharoor with Rachel Pannett. How ancient India changed the world. WorldView (by mail). washingtonpost.com. May 19, 2025.

https://s2.washingtonpost.com/camp-rw/?trackId=660de34bb906a33aae6b8103&s=682aadf6f062960b82a2f3e6

Also:  forms of power, waves, attractor, Zen, compassion, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: life, forms of power, soft power, waves, attractors, Zen, compassion, transition.

# life: Balzac's world revisited

<< In a recent study picked up in the French press, the academic Mélanie Plouviez cites one of her country’s best-loved novelists to make a damning point. The power of inherited and unearned wealth in the France of 2025, she argues, replicates the social injustices found in Honoré de Balzac’s 19th-century chronicles of ambition and despair. As in the 1820s, she writes, “Who now could buy a place in Paris relying only on their wage and without family help? With the resurgence of inherited wealth, a gulf between what work allows and inheritance allows has also returned.” >>

<< The problem is a sadly familiar one across Europe, and the same observation could be made of Britain, Germany or Italy. >>

Editorial. The Guardian view on Europe’s growing wealth divide: back to the world of Balzac. A new study highlights the dangers of a modern rentier capitalism that perpetuates inequality through the generations. The Guardian. Thu 15 May 2025 19.25 CEST.

https://www.theguardian.com/commentisfree/2025/may/15/the-guardian-view-on-europes-growing-wealth-divide-back-to-the-world-of-balzac

Also: oops! AI - artificial intelligence - now beats humans at basic tasks. FonT. Apr 17, 2024. 

https://flashontrack.blogspot.com/2024/04/life-oops-ai-artificial-intelligence.html

Also: ai (artificial intell) (bot), in https://www.inkgmr.net/kwrds.html 

Keywords: life, ai, artificial intelligence, bot, income, ubi, unconditional basic income

Font: here is an intriguing question: who knows what any State will ask of individual citizens in exchange for the essential Citizenship allowance (e.g.Unconditional Basic Income, UBI), an allowance that it will have to give to the entire population to stabilize the survival of any community.

# gst: emergent scattering regimes in disordered metasurfaces near critical packing.

<< Disordered metasurfaces provide a versatile platform for harnessing near- and far-field scattered light. Most research has focused on either particulate topologies composed of individual, well-identified metaatoms or, to a lesser extent, semi-continuous aggregate topologies without well identified inclusions. >>

Here, AA << uncover an intermediate critical packing regime characterized by metasurface morphologies in which a significant fraction of metaatoms begin to connect. (They) experimentally demonstrate that, at this threshold, the properties of the scattered light abruptly change and interpret this change as a marked transition in the statistics of the photon density of states. >>

<< Unlike percolation metal films, this transition affects not only the specular but also the diffuse components of the scattered light in a profound way. (AA)  results introduce critical packing topologies as a novel design strategy for manipulating the spectral and angular characteristics of light using ultrathin optical coatings. Emergent functionalities include color shifts in diffuse light driven by multiple scattering and surface whitening, >>

Miao Chen, Adrian Agreda, et al. Emergent scattering regimes in disordered metasurfaces near critical packing. arXiv: 2505.02244v1 [physics.optics]. May 4, 2025.

https://arxiv.org/abs/2505.02244

Also: disorder & fluctuations, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, disorder, fluctuations, transitions, disordered metasurfaces, ultrathin optical coatings, near- far- field scattered light, critical packing regime.

# gst: elasticity of fibers prefers the chaos of turbulence.

<< The dynamics of fibers, modeled as a sequence of inertial beads linked via elastic springs, in turbulent flows is dictated by a nontrivial interplay of inertia and elasticity. Such elastic, inertial fibers preferentially sample a three-dimensional turbulent flow in a manner that is qualitatively similar to that in two dimensions [R. Singh et al., Phys. Rev. E 101, 053105 (2020)]. >>

<< Both these intrinsic features have competing effects on fiber dynamics: Inertia drives fibers away from vortices while elasticity tends to trap them inside. However, these effects swap roles at very large values. A large inertia makes the fibers sample the flow more uniformly while a very large elasticity facilitates the sampling of straining regions. >>

<< This complex sampling behavior is further corroborated by quantifying the chaotic nature of sampled flow regions. This is achieved by evaluating the maximal Lagrangian Lyapunov Exponents associated with the flow along fiber trajectories. >>

Rahul K. Singh. Elasticity of fibers prefers the chaos of turbulence. Phys. Rev. E 111, L053101. May 5, 2025.

https://journals.aps.org/pre/abstract/10.1103/PhysRevE.111.L053101

Also: elastic, turbulence, chaos, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, elasticity, turbulence, chaos, transitions

# gst: hyperchaos and complex dynamical regimes in N-d neuron lattices.

AA << study the dynamics of N-dimensional lattices of nonchaotic Rulkov neurons coupled with a flow of electrical current. (They) consider both nearest-neighbor and next-nearest-neighbor couplings, homogeneous and heterogeneous neurons, and small and large lattices over a wide range of electrical coupling strengths. >>

<< As the coupling strength is varied, the neurons exhibit a number of complex dynamical regimes, including unsynchronized chaotic spiking, local quasi-bursting, synchronized chaotic bursting, and synchronized hyperchaos. >>

<< For lattices in higher spatial dimensions, (AA) discover dynamical effects arising from the ``destructive interference'' of many connected neurons and miniature ``phase transitions'' from coordinated spiking threshold crossings. In large two- and three-dimensional neuron lattices, (They) observe emergent dynamics such as local synchronization, quasi-synchronization, and lag synchronization. >>

<< These results illustrate the rich dynamics that emerge from coupled neurons in multiple spatial dimensions, highlighting how dimensionality, connectivity, and heterogeneity critically shape the collective behavior of neuronal systems. >>

Brandon B. Le, Dima Watkins. Hyperchaos and complex dynamical regimes in N-dimensional neuron lattices. arXiv: 2505.03051v1 [nlin.CD]. May 5, 2025.

https://arxiv.org/abs/2505.03051

Also: brain, network, behavior, chaos, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, brain, network, behavior, cooperation, cooperative behavior, chaos, hyperchaos, transitions, phase transitions, transition thresholds,  synchrony, dimensionality, topology of connectivity, intermittent bursting activity, interference, destructive interference.

# gst: overcoming overly simplistic representations, chaos and regularity in an anisotropic soft squircle billiard.

<< A hard-wall billiard is a mathematical model describing the confinement of a free particle that collides specularly and instantaneously with boundaries and discontinuities. >>

<< Soft billiards are a generalization that includes a smooth boundary whose dynamics are governed by Hamiltonian equations and overcome overly simplistic representations. >>

AA << study the dynamical features of an anisotropic soft-wall squircle billiard. This curve is a geometric figure that seamlessly blends the angularity of a square with the smooth curves of a circle. (AA) characterize the billiard's emerging trajectories, exhibiting the onset of chaos and its alternation with regularity in the parameter space. (They) characterize the transition to chaos and the stabilization of the dynamics by revealing the nonlinearity of the parameters (squarness, ellipticity, and hardness) via the computation of Poincaré surfaces of section and the Lyapunov exponent across the parameter space. >>

AA << expect (Their) work to introduce a valuable tool to increase understanding of the onset of chaos in soft billiards. >>

A. González-Andrade, H. N. Núñez-Yépez, M. A. Bastarrachea-Magnani. Chaos and Regularity in an Anisotropic Soft Squircle Billiard. arXiv: 2504.20270v1 [nlin.CD]. Apr 28, 2025.

https://arxiv.org/abs/2504.20270

Also: billiard, chaos, particle, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, billiard, soft billiard, soft-wall squircle billiard, particles, smooth boundary,  specular collisions, transitions, chaos

# gst: apropos of critical transitions, a new approach to extreme events.

FIG. 1. Dynamics of excitable complex networks [coupling topologies: random (RN); small-world (SW); scale-free (SF); all-to-all (complete; CP)]. 

<< Unexpected and often irreversible shifts in the state or the dynamics of a complex system often accumulate in extreme events with likely disastrous impact on the system and its environment. Detection, understanding, and possible prediction of such critical transitions are thus of paramount importance across a variety of scientific fields. >>

<< The rather modest improvement achieved so far may be due previous research mostly concentrating on either particular subsystems, considered to be of vital importance for the generating mechanism of a critical transition, or on the system as a whole. These approaches only rarely take into account the intricate, time-dependent interrelatedness of subsystems that can essentially determine emerging behaviors underlying critical transitions. >> 

AA << uncover subsystems, network vertices, and the interrelatedness of certain subsystems, network edges, as tipping elements in a networked dynamical system, forming a time-evolving tipping subnetwork. (They)  demonstrate the existence of tipping subnetworks in excitable complex networks and in human epileptic brains. These systems can repeatedly undergo critical transitions that result in extreme events. >>

AA << findings reveal that tipping subnetworks encapsulate key properties of mechanisms involved in critical transitions. >>

Timo Bröhl, Klaus Lehnertz. Emergence of a tipping subnetwork during a critical transition in networked systems: A new avenue to extreme events. Phys. Rev. Research 7, 023109. May 1, 2025.

https://journals.aps.org/prresearch/abstract/10.1103/PhysRevResearch.7.023109

Also: network, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, networks, excitable complex networks, network edges, network vertices, subnetwork, tipping subnetworks, small-worlds, unexpected shifts, transitions, critical transition, extreme events, interrelatedness, time-dependent interrelatedness.

# gst: emergent oscillations and chaos in noncompliant microfluidic networks.

<< Incompressible fluids in microfluidic networks with nonrigid channels can exhibit flow rate oscillations analogous to electric current oscillations in RLC (resistor, inductor, capacitor) circuits. This is due to the elastic deformation of channel walls that can store and release fluid, as electric capacitors can store and release electric charges. This property is quantified through the compliance of the system, defined as the volume change relative to the pressure change. >>

<< In systems with rigid walls and incompressible fluid, compliance vanishes, and no oscillations can occur through this mechanism. >>

Here, AA << show that not only oscillations but also chaos can emerge in the flow-rate dynamics of noncompliant microfluidic networks with incompressible fluid. Notably, these dynamics emerge spontaneously, even under time-independent driving pressures. The underlying mechanism is governed by the effect of fluid inertia, which becomes relevant at moderate Reynolds numbers observed in microfluidic systems exhibiting complex flow patterns. >>

<< The results are established using a combination of direct numerical simulations and a reduced model derived from modal analysis. This approach enables (AA) to determine the onset of oscillations, the associated bifurcations, the oscillation frequencies and amplitudes, and their dependence on the driving pressures. >>

Yanxuan Shao, Jean-Regis Angilella, Adilson E. Motter. Emergent oscillations and chaos in noncompliant microfluidic networks. Phys. Rev. Fluids 10, 054401. May 1, 2025.

https://journals.aps.org/prfluids/abstract/10.1103/PhysRevFluids.10.054401

arXiv: 2505.00068v1 [physics.flu-dyn]. 

https://arxiv.org/abs/2505.00068

Also: network, elastic, chaos, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, networks, microfluidic networks, noncompliant networks with incompressible fluid, fluid inertia, 

driving pressures, elasticity, chaos.

# gst: apropos of adaptation of simple organisms to changing environments, self-organization and memory in a disordered entity to random driving.

AA << consider self-organization and memory formation in a mesoscopic model of an amorphous solid subject to a protocol of random shear confined to a strain range ±𝜖max. (They) develop proper readout protocols to show that the response of the driven system self-organizes to retain a memory of the strain range, which can be subsequently retrieved. >>

AA << findings generalize previous results obtained upon oscillatory driving and suggest that self-organization and memory formation of disordered materials can emerge under more general conditions, such as a disordered system interacting with its fluctuating environment. Self-organization results in a correlation between the dynamics of the system and its environment, providing thereby an elementary mechanism for sensing. >>

AA << conclude by discussing (Their)  results and their potential relevance for the adaptation of simple organisms lacking a brain to changing environments. >>

Muhittin Mungan, Dheeraj Kumar, et al. Self-Organization and Memory in a Disordered Solid Subject to Random Driving. Phys. Rev. Lett. 134, 178203. April 30, 2025.

https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.134.178203

arXiv: 2409.17096v2 [cond-mat.soft]. 

https://arxiv.org/abs/2409.17096

Also: disorder & fluctuations, 

self-assembly, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, disorder, fluctuations, self-assembly, self-organization, transitions

# gst: inverse design of Kirigami; contracted shapes, deployed shapes, internal trajectories of rotating units.

<< Kirigami metamaterials have enabled a plethora of morphing patterns across art and engineering. However, the inverse design of kirigami for complex shapes remains a puzzle that so far cannot be solved without relying on complex numerical methods. >>

Here, AA << present a purely geometric design method to overcome the reliance on sophisticated numerical algorithms and showcase how to leverage it for three distinct types of morphing targets, i.e., the contracted shape, the deployed shape, and the internal trajectories of the rotating units in kirigami specimens. >>

AA << results unveil the fundamental relations between the kirigami deformation and the shape of its rotating units and enable us to establish the underpinning physics through theoretical investigations validated via numerical simulations. >>

Chuan Qiao, Shijun Chen, et al. Inverse Design of Kirigami through Shape Programming of Rotating Units. Phys. Rev. Lett. 134, 176103. May 2, 2025.

https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.134.176103

Also: kirigami, origami, metamorphosis,  in https://www.inkgmr.net/kwrds.html 

Keywords: gst, kirigami, origami, metamorphosis

# gst: like fireflies or neurons, dynamics of pulsating swarmalators on a ring.

AA << study a simple one-dimensional model of swarmalators, a generalization of phase oscillators that swarm around in space as well as synchronize internal oscillations in time. Previous studies of the model focused on Kuramoto-type couplings, where the phase interactions are governed by phase differences. >>

 Here AA << consider Winfree-type coupling, where the interactions are multiplicative, determined by the product of a phase response function  and phase pulse function . This more general interaction (from which the Kuramoto phase differences emerge after averaging) produces rich physics: six long-term modes of organization are found, which we characterize numerically and analytically. >>

Samali Ghosh, Kevin O'Keeffe, et al. Dynamics of pulsating swarmalators on a ring. arXiv: 2504.14912v1 [nlin.AO]. Apr 21, 2025. 

https://arxiv.org/abs/2504.14912

Also: swarm, swarmalators, instability, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, swarm, swarmalators, instability

# gst: transitions of breakup regimes for viscous droplets in airflow.

In this AA study, << the transitions of breakup regimes for viscous droplets are investigated experimentally using high-speed imaging taken from a side view and a 45 view. Based on the morphology change in the middle of the droplet, the breakup regimes are classified into no-breakup, bag breakup, bag-stamen, low-order multimode, high-order multimode, and shear-stripping breakup. The droplet morphologies in different regimes and the corresponding transitions are discussed in detail. >>

<< The droplet viscosity dissipates the kinetic energy transferred by the airflow during the initial droplet flattening, and affects the development of the Rayleigh-Taylor instability wave after the flattening. Through the analysis of the droplet deformation and the Rayleigh-Taylor instability with the droplet viscosity taken into account, the transition conditions of different regimes are obtained in a regime map. By further considering the relative velocity loss between the droplet and the airflow, the ranges of the dual-bag breakup in the low-order multimode regime and the droplet retraction in the bag-stamen regime are determined. >>

Zhikun Xu, Tianyou Wang, Zhizhao Che. Transitions of breakup regimes for viscous droplets in airflow. arXiv: 2504.14149v1 [physics.flu-dyn]. Apr 19, 2025.

https://arxiv.org/abs/2504.14149

Also: drop, droplet, droploid, transition, instability, waves, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, drops, droplets, droploids, viscous droplets, droplet breakup, transitions, instability, waves

# gst: period-doubling route to chaos in viscoelastic flows

<< Polymer solutions can develop chaotic flows, even at low inertia. This purely elastic turbulence is well studied, but little is known about the transition to chaos. In two-dimensional (2D) channel flow and parallel shear flow, traveling wave solutions involving coherent structures are present for sufficiently large fluid elasticity. >>

AA << numerically study 2D periodic parallel shear flow in viscoelastic fluids, and (They) show that these traveling waves become oscillatory and undergo a series of period-doubling bifurcations en-route to chaos. >>

Jeffrey Nichols, Robert D. Guy, Becca Thomases. Period-doubling route to chaos in viscoelastic Kolmogorov flow. Phys. Rev. Fluids 10, L041301. Apr 17, 2025.

https://journals.aps.org/prfluids/abstract/10.1103/PhysRevFluids.10.L041301

Also: chaos, waves, elastic, turbulence, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, chaos, waves, traveling waves, elasticity, viscoelastic fluids, turbulence, elastic turbulence, period-doubling bifurcations, transitions