# gst: criticality and multistability in quasi-2D turbulence

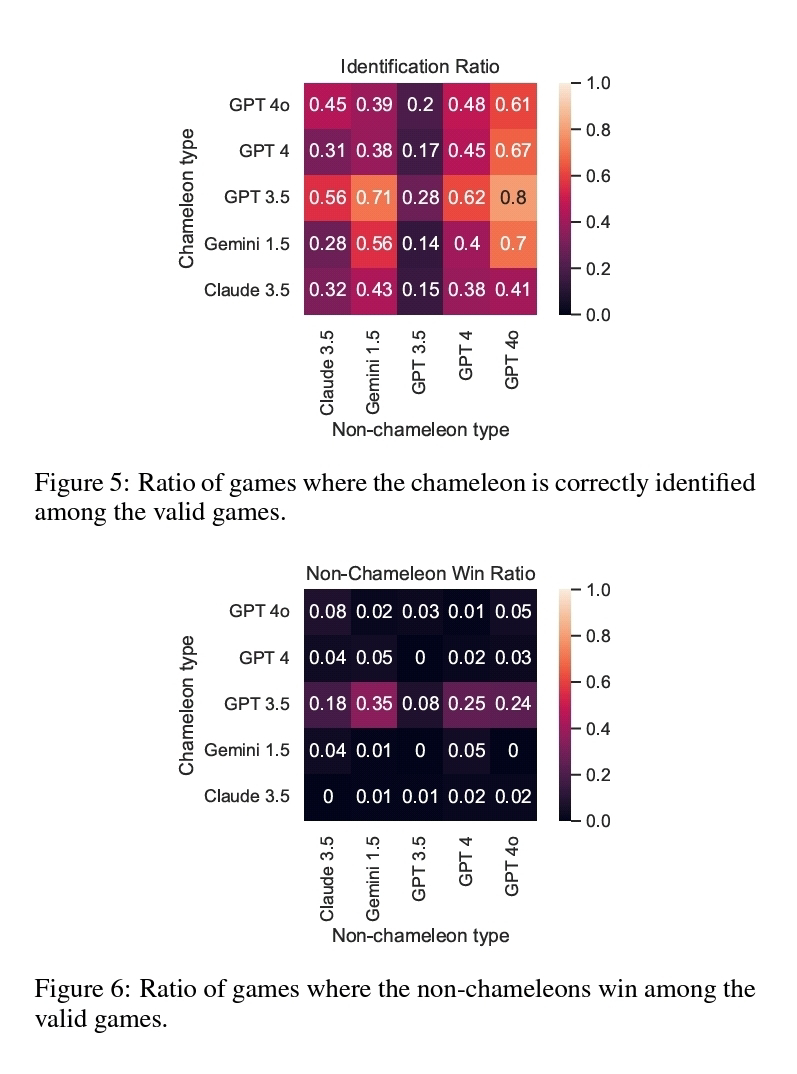
       Fig. 1(a) Helmholtz resonators

<< Two-dimensional (2D) turbulence, despite being an idealization of real flows, is of fundamental interest as a model of the spontaneous emergence of order from chaotic flows. The emergence of order often displays critical behavior, whose study is hindered by the long spatial and temporal scales involved. >>

Here AA << experimentally study turbulence in periodically driven nanofluidic channels with a high aspect ratio using superfluid helium. (They) find a multistable transition behavior resulting from cascading bifurcations of large-scale vorticity and critical behavior at the transition to quasi-2D turbulence consistent with phase transitions in periodically driven many-body systems. >>

AA << demonstrate that quasi-2D turbulent systems can undergo an abrupt change in response to a small change in a control parameter, consistent with predictions for large-scale atmospheric or oceanic flows. >>️

Filip Novotny, Marek Talir, et al. Critical behavior and multistability in quasi-two-dimensional turbulence. arXiv: 2406.08566v1 [physics.flu-dyn]. Jun 12, 2024.

https://arxiv.org/abs/2406.08566

Also: order, disorder, disorder & fluctuations, turbulence, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, order, disorder, disorder & fluctuations, criticality, turbulence, transition 

# life: spontaneous emergence of run-and-tumble-like dynamics in coupled self-propelled bots.

<< Drawing inspiration from the motility behaviour of microorganisms, we introduce a highly tunable, robotic system self-actuating into the run-and-tumble (RT)-like motion. It comprises two disk-shaped, centimeter-scale programmable robots individually programmed to perform overdamped active Brownian (AB) motion and connected by a rigid rod. The rod is attached to pivot points located on off-centered, mirror-symmetric points on each robot, allowing for its free rotation at both ends. >>

AA << show that the collective dynamics of this system execute RT-like motion with characteristic sharp tumble events and exponentially distributed run times, similar to those observed in microorganisms. (They) further quantify emerging dynamics in terms of tumbling frequency and tune it over a wide range of experimental parameters. >>

AA << also develop a theoretical model that reproduces our experimental results and elucidates the underlying physical mechanisms governing the rich phase behavior of RT motion. >>

 ️

Somnath Paramanick, Umashankar Pardhi, et al. Spontaneous emergence of run-and-tumble-like dynamics in coupled self-propelled robots: experiment and theory. arXiv: 2502.01257v1 [cond-mat.soft]. Feb 3, 2025.

https://arxiv.org/abs/2502.01257

Also: behav, evolution, bot, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, life, behavior, evolution, bots, self-propelled bots, run-and-tumble motion 

# life: chameleon machines

<< Large language model-based (LLM-based) agents have become common in settings that include non-cooperative parties. In such settings, agents' decision-making needs to conceal information from their adversaries, reveal information to their cooperators, and infer information to identify the other agents' characteristics. To investigate whether LLMs have these information control and decision-making capabilities, (AA) make LLM agents play the language-based hidden-identity game, The Chameleon. >>️

<< Based on the empirical results and theoretical analysis of different strategies, (AA) deduce that LLM-based non-chameleon agents reveal excessive information to agents of unknown identities. (Their) results point to a weakness of contemporary LLMs, including GPT-4, GPT-4o, Gemini 1.5, and Claude 3.5 Sonnet, in strategic interactions. >>

️

Mustafa O. Karabag, Ufuk Topcu. Do LLMs Strategically Reveal, Conceal, and Infer Information? A Theoretical and Empirical Analysis in The Chameleon Game. arXiv: 2501.19398v1 [cs.AI]. Jan 31, 2025.

https://arxiv.org/abs/2501.19398

Also: games, ai (artificial intell), nfulaw, in https://www.inkgmr.net/kwrds.html 

Keywords: life, games, chameleon game, ai, artificial intelligence, LLMs, privacy, nfulaw

# gst: discontinuous transitions to active nematic turbulence.

<< Active fluids exhibit chaotic flows at low Reynolds number known as active turbulence. Whereas the statistical properties of the chaotic flows are increasingly well understood, the nature of the transition from laminar to turbulent flows as activity increases remains unclear. Here, through simulations of a minimal model of unbounded active nematics, (AA) find that the transition to active turbulence is discontinuous. (They) show that the transition features a jump in the mean-squared velocity, as well as bistability and hysteresis between laminar and chaotic flows. >>

<< From distributions of finite-time Lyapunov exponents, (AA) identify the transition at a value A∗≈4900 of the dimensionless activity number. Below the transition to chaos, (They) find subcritical bifurcations that feature bistability of different laminar patterns. These bifurcations give rise to oscillations and to chaotic transients, which become very long close to the transition to turbulence. Overall, (Their) findings contrast with the continuous transition to turbulence in channel confinement, where turbulent puffs emerge within a laminar background. >>

AA << propose that, without confinement, the long-range hydrodynamic interactions of Stokes flow suppress the spatial coexistence of different flow states, and thus render the transition discontinuous. >>️

Malcolm Hillebrand, Ricard Alert. Discontinuous Transition to Active Nematic Turbulence. arXiv: 2501.06085v1 [cond-mat.soft]. Jan 10, 2025.

https://arxiv.org/abs/2501.06085

Also: chaos, transition, turbulence, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, chaos, transition, turbulence, jumps, active nematics

# gst: quadrupolar stress drives collapse of nematic order on frictional substrates.

<< The field of active nematics has traditionally employed descriptions based on dipolar activity, with interactions that align along a single axis. However, it has been theoretically predicted that interactions with a substrate, prevalent in most biological systems, would require novel forms of activity, such as quadrupolar activity, that are governed by hydrodynamic screening. >>

<< Here, by combining experiments and numerical simulations, (AA) show that upon light-induced solidification of the underlying medium, microtubule-kinesin mixtures undergo a transformation that leads to a biphasic active suspension. Using an active lyotropic model, (They) prove that the transition is governed by screening effects that alter the dominant form of active stress. Specifically, the combined effect of friction and quadrupolar activity leads to a hierarchical folding that follows the intrinsic bend instability of the active nematic layer. >>

AA << results demonstrate the dynamics of the collapse of orientational order in active nematics and present a new route for controlling active matter by modifying the activity through changing the surrounding environment. >>️

Aleksandra Ardaseva, Ignasi Velez-Ceron, et al. Beyond Dipolar Activity: Quadrupolar Stress Drives Collapse of Nematic Order on Frictional Substrates. arXiv: 2407.03723v3 [cond-mat.soft]. Jan 14, 2025. 

https://arxiv.org/abs/2407.03723

Also: transition, collapse, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, transition, collapse, active nematics, stress 

# gst: limit cycles and chaos in planar hybrid systems.

<< The main inspiration of (this AA) work is the paper of Llibre and Teixeira (Nonlinear Dyn. 91, No. 1, 249-255, 2018) about Filippov systems formed by two linear centers and having x = 0 as discontinuity line. One of the main conclusions of the paper is that such systems cannot have limit cycles. Actually, either it does not have periodic orbits or every orbit is periodic. Therefore, its dynamics is relatively simple. Inspired by this work and the raising notion of hybrid systems, (AA) wondered what could happen if we allow jumps on the discontinuity line. As a result, (They) discovered not only that limit cycles are allowed with arbitrarily small “perturbations” in the jump, (..), but also that such systems allow chaotic dynamics. Therefore, (AA) conclude that hybrid systems with simple formulation can have rich dynamics. (They) also observe that a complete characterization of the dynamics of X ∈ Xn depends on the characterization of its first return map, which is a piecewise polynomial map on the real line. This, together with the fact that the systems studied here are a generalization of the Filippov systems (..), illustrates that hybrid systems can be seen as a three-fold bridge connecting continuous, piecewise continuous and discrete dynamical systems. >>️

Jaume Llibre, Paulo Santana. Limit cycles and chaos in planar hybrid systems. arXiv: 2407.05151v2 [math.DS]. Oct 1, 2024. 

https://arxiv.org/abs/2407.05151

Also: chaos, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, limit cycles, chaos, transitions, small perturbations, jumps  

# gst: instability, shocks, and competition interfaces in the Brownian last-passage percolation model

<<  For stochastic Hamilton-Jacobi (SHJ) equations, instability points are the space-time locations where two eternal solutions with the same asymptotic velocity differ. Another crucial structure in such equations is shocks, which are the space-time locations where the velocity field is discontinuous. >>

AA << provide a detailed analysis of the structure and relationships between shocks, instability, and competition interfaces in the Brownian last-passage percolation model, which serves as a prototype of a semi-discrete inviscid stochastic HJ equation in one space dimension. >>

AA << show that the shock trees of the two unstable eternal solutions differ within the instability region and align outside of it. Furthermore, (They) demonstrate that one can reconstruct a skeleton of the instability region from these two shock trees. >>️

Firas Rassoul-Agha, Mikhail Sweeney. Shocks and instability in Brownian last-passage percolation. arXiv:  2407.07866v2 [math.PR]. Oct 18, 2024. 

https://arxiv.org/abs/2407.07866

Also: Brownian last-passage percolation (LPP) model. In: S. GANGULY,  A. HAMMOND. Stability and chaos in dynamical last passage percolation. Jun 7, 2024. 

 https://doi.org/10.1090/cams/35 

Keywords: gst, instability, shock, competition, chaos, percolation