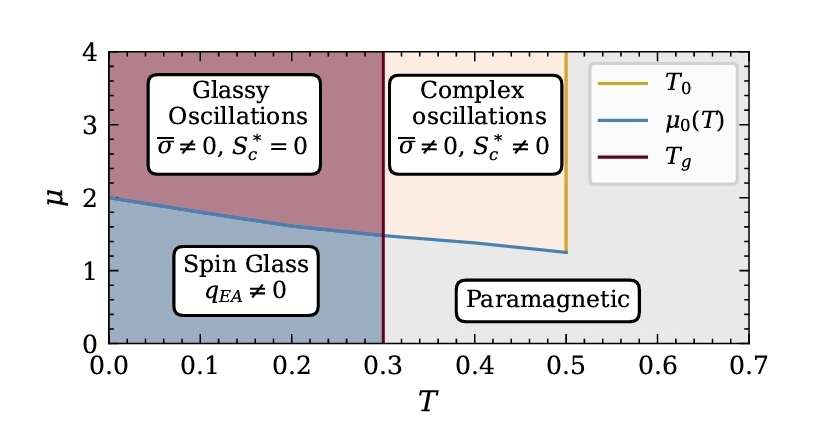
<< ️In many active systems, swimmers collectively stir the surrounding fluid to stabilize some self-sustained vortices. The resulting nonequilibrium state is often referred to as active turbulence, by analogy with the turbulence of passive fluids under external stirring. Although active turbulence clearly operates far from equilibrium, it can be challenging to pinpoint which emergent features primarily control the deviation from an equilibrium reversible dynamics. >>
Here, AA << reveal that dynamical irreversibility essentially stems from singularities in the active stress. Specifically, considering the coupled dynamics of the swimmer density and the stream function, (AA) demonstrate that the symmetries of vortical flows around defects determine the overall irreversibility. (Their) detailed analysis leads to identifying specific configurations of defect pairs as the dominant contribution to irreversibility. >>
Byjesh N. Radhakrishnan, Francesco Serafin, et al. Irreversibility in scalar active turbulence: The role of topological defects. arXiv:2507.06073v1 [cond-mat.stat-mech]. Jul 8, 2025.
Also: swim, turbulence, vortex, self-assembly, chaos, clinamen, in https://www.inkgmr.net/kwrds.html
Keywords: gst, swim, swimmers, turbulence, active turbulence, vortex, self-assembly, chaos, defects, topological defects, deviation, clinamen.