# gst: apropos of Parrondo paradox, controlling quantum chaos via Parrondo strategies on noisy intermediate-scale quantum hardware

<< ️Advancements in noisy intermediate-scale quantum (NISQ) computing are steadily pushing these systems toward outperforming classical supercomputers on specific well-defined computational tasks. In this work (AA) explore and control quantum chaos in NISQ systems using discrete-time quantum walks (DTQWs) on cyclic graphs. To efficiently implement quantum walks on NISQ hardware, (They) employ the quantum Fourier transform to diagonalize the conditional shift operator, optimizing circuit depth and fidelity. >>

<< ️(AA) experimentally realize the transition from quantum chaos to order via DTQW dynamics on both odd and even cyclic graphs, specifically 3- and 4-cycle graphs, using the counterintuitive Parrondo paradox strategy across three different NISQ devices. >>

<< ️While the 4-cycle graphs exhibit high-fidelity quantum evolution, the 3-cycle implementation shows significant fidelity improvement when augmented with dynamical decoupling pulses. (Their) results demonstrate a practical approach to probing and harnessing controlled chaotic dynamics on real quantum hardware, laying the groundwork for future quantum algorithms and cryptographic protocols based on quantum walks. >>

Aditi Rath, Dinesh Kumar Panda, Colin Benjamin. Controlling quantum chaos via Parrondo strategies on noisy intermediate-scale quantum hardware. Phys. Rev. E 112, 054219. Nov 18, 2025.

https://journals.aps.org/pre/abstract/10.1103/m89r-2dy5

arXiv: 2506.11225v2 [quant-ph]. Nov 4, 2025.

https://arxiv.org/abs/2506.11225

Also: parrondo, noise, walk, walking, order, chaos, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, parrondo, noise, walk, walking, quantum walk, order, chaos, quantum chaos, transition, dynamical decoupling pulses, cryptography.

# gst: apropos of itinerant behaviors, from chaotic itinerancy to intermittent synchronization in complex networks.

<< ️Although synchronization has been extensively studied, important processes underlying its emergence have remained hidden by the use of global order parameters. Here, (AA) uncover how the route unfolds through a sequential transition between two well-known but previously unconnected phenomena: chaotic itinerancy (CI) and intermittent synchronization (IS). >>

<< ️Using a new symbolic dynamics, (They) show that CI emerges as a collective yet unsynchronized exploration of different domains of the high-dimensional attractor, whose dimension is reduced as the coupling increases, ultimately collapsing back into the reference chaotic attractor of an individual unit. At this stage, the IS can emerge as irregular alternations between synchronous and asynchronous phases. The two phenomena are therefore mutually exclusive, each dominating a distinct coupling interval and governed by different mechanisms. >>

<< ️Network structural heterogeneity enhances itinerant behavior since access to different domains of the attractor depends on the nodes' topological roles. The CI--IS crossover occurs within a consistent coupling interval across models and topologies. Experiments on electronic oscillator networks confirm this two-step process, establishing a unified framework for the route to synchronization in complex systems. >>

I. Leyva, Irene Sendiña-Nadal, Christophe Letellier, et al. From chaotic itinerancy to intermittent synchronization in complex networks. arXiv: 2511.09253v1 [nlin.AO]. Nov 12, 2025.

https://arxiv.org/abs/2511.09253

Also: network, behav, intermittency, transition, attractor, chaos, collapse, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, networks, behavior, intermittency, transitions, attractor, chaos, collapse, chaotic itinerancy, intermittent synchronization, structural heterogeneity, itinerant behavior.

# gst: effect of stochasticity on initial transients and chaotic itinerancy for a natural circulation loop.

<< ️The introduction of stochastic forcing to dynamical systems has been shown to induce qualitatively different behaviors, such as attractor hopping, to otherwise stable systems as they approach bifurcation. In this (AA) study, the effect of stochastic forcing on systems that have already undergone bifurcation and evolve on a chaotic attractor is explored. Markov and state-independent models of turbulence-induced stochasticity are developed, and their effects on a natural circulation loop operating in the chaotic regime are compared. >>

<< ️Stochasticity introduces considerable uncertainty into the duration of the initial chaotic transient but tends to accelerate it on average. An Ornstein-Uhlenbeck model of turbulent fluctuations is shown to produce results equivalent to a bootstrapped raw direct numerical simulation signal. >>

<< Similar, though less pronounced, effects are found for systems operating in the chaotic itinerant regime. The Markov model of chaotic itinerancy which is typically applied to this class of problems is shown to be invalid for this system and the Lorenz system, to which it has been applied in the past. >>

<< ️Off-discrete transitions and an upper limit on the time between flow reversals are explained by near misses of the attractor ruins caused by lingering excitation of high-order modes during chaotic itinerancy. >>

John Matulis, Hitesh Bindra. Effect of stochasticity on initial transients and chaotic itinerancy for a natural circulation loop. Phys. Rev. E 112, 044223. Oct 23, 2025

https://journals.aps.org/pre/abstract/10.1103/42pn-wttx

Also: disorder & fluctuations, turbulence, attractor, chaos, transition, uncertainty, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, disorder, fluctuations, turbulence, attractor, chaos, transition, uncertainty, stochasticity, flow instability, chaotic itinerancy, noise-induced transitions.

# gst: implementation of a generalized intermittency scenario in the Rossler dynamical system.

<< The realization of novel scenario involving transitions between different types of chaotic attractors is investigated for the Rossler system. Characteristic features indicative of the presence of generalized intermittency scenario in this system are identified. The properties of "chaos-chaos" transitions following the generalized intermittency scenario are analyzed in detail based on phase-parametric characteristics, Lyapunov characteristic exponents, phase portraits, and Poincare sections. >>

O.O. Horchakov, A.Yu. Shvets. Implementation of a generalized intermittency scenario in the Rossler dynamical system. arXiv: 2511.03364v1 [nlin.CD]. Nov 5, 2025.

https://arxiv.org/abs/2511.03364

Also: intermittency, attractor, chaos, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, intermittency, attractors, chaos, transitions, chaos-chaos transitions.

# gst: energy transport and chaos in a one-dimensional disordered nonlinear stub lattice

<< ️(AA) investigate energy propagation in a one-dimensional stub lattice in the presence of both disorder and nonlinearity. In the periodic case, the stub lattice hosts two dispersive bands separated by a flat band; however, (They) show that sufficiently strong disorder fills all intermediate band gaps. By mapping the two-dimensional parameter space of disorder and nonlinearity, (AA) identify three distinct dynamical regimes (weak chaos, strong chaos, and self-trapping) through numerical simulations of initially localized wave packets. >>

<< ️When disorder is strong enough to close the frequency gaps, the results closely resemble those obtained in the one-dimensional disordered discrete nonlinear Schrödinger equation and Klein-Gordon lattice model. In particular, subdiffusive spreading is observed in both the weak and strong chaos regimes, with the second moment m_2 of the norm distribution scaling as m_2 ∝ t^0.33 and m_2 ∝ t^0.5, respectively. The system’s chaotic behavior follows a similar trend, with the finite-time maximum Lyapunov exponent Λ decaying as Λ ∝ t^−0.25 and Λ ∝ t^−0.3. For moderate disorder strengths, i.e., near the point of gap closing, (They) find that the presence of small frequency gaps does not exert any noticeable influence on the spreading behavior. >>

<< ️(AA) findings extend the characterization of nonlinear disordered lattices in both weak and strong chaos regimes to other network geometries, such as the stub lattice, which serves as a representative flat-band system. >>

Su Ho Cheong, Arnold Ngapasare, et al. Energy transport and chaos in a one-dimensional disordered nonlinear stub lattice. arXiv: 2511.04159v1 [nlin.CD].  Nov 6, 2025.

https://arxiv.org/abs/2511.04159

Also: network, waves, disorder, chaos, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, networks, waves, disorder, chaos, stub lattice, subdiffusive spreading.

# gst: transition to chaos with conical billiards.

<< ️In this paper, (AA) introduced and extensively investigated dynamical billiards on the surface of a cone with a tilted base. Upon varying the cone angle β, corresponding to a deficit angle 

2πχ = 2π(1 − sin(β)), and tilt angle γ, (They) identified three distinct types of trajectories with associated Poincaré map for conical billiards: rim, hourglass, and mixed. >>

<< ️Region I, where Poincaré space consists of rim, hourglass, and mixed trajectories; Region IIB, where Poincaré space consists of only hourglass and mixed trajectories; and Region IIA, in which (They) find choices of γ and χ for which almost all trajectories are strongly mixing. (..) (AA) also developed a scheme for identifying strongly mixing trajectories. >>

<< ️Furthermore, (They) were able to show that a dynamical billiard on a surface with exclusively convex and positive Gaussian curvature in three dimensions can still exhibit ergodic behavior in certain parameter regimes. >>

<< ️A particularly intriguing feature of this system is that by tuning χ and γ, nearly all points in (θ,ϕ) Poincaré space describing conical line segments in between bounces can be placed at the edge between chaotic and integrable dynamics. Thus this work highlights the potential of conical billiards as a model system for exploring intriguing problems inspired by neural networks at the “edge of chaos”. >>

Lara Braverman, David R. Nelson. Transition to chaos with conical billiards. arXiv: 2508.02786v1 [nlin.CD]. Aug 4, 2025. 

https://arxiv.org/abs/2508.02786

Phys. Rev. E 112, 044221. Oct 21, 2025.

https://journals.aps.org/pre/abstract/10.1103/18f7-sqhz

Also: billiard, chaos, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, billiards, conical billiard, chaos, transitions, neural networks

# ecol: stabilization of macroscopic dynamics by fine-grained disorder in many-species ecosystems.

<< ️Models for complex, heterogeneous systems such as ecological communities vary in the level of organization they describe. When the dynamics at the species level is unknown, one typically resorts to heuristic "macroscopic" models that capture relationships among a few degrees of freedom, e.g., groups of similar species, and commonly display out-of-equilibrium dynamics. These models, however, exactly reflect the species-level "microscopic" dynamics only when microscopic heterogeneity can be neglected. >>

<< ️Here, (AA) address the robustness of such macroscopic descriptions to the addition of disordered microscopic interactions. While disorder is known to destabilize equilibria at the microscopic level, leading to asynchronous (typically chaotic) fluctuations, (They) show that it can also stabilize synchronous species fluctuations driven by macroscopic structure. >>

<< ️(AA) analytically find the conditions for the existence of heterogeneity-stabilized equilibria and relate their stability to a mismatch in the time scales of individual species. This may shed light on the empirical observation that many-species ecosystems often appear stable despite highly diverse and potentially destabilizing interactions between species and functional groups. >>

Juan Giral Martínez, Silvia De Monte, Matthieu Barbier. Stabilization of macroscopic dynamics by fine-grained disorder in many-species ecosystems. Phys. Rev. E 112, 034305. Sep 4, 2025.

https://journals.aps.org/pre/abstract/10.1103/5w5x-b1c2

Also: disorder, disorder & fluctuations, chaos, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, disorder, disorder & fluctuations, chaos, ecological communities, disordered microscopic interactions, asynchronous fluctuations, heterogeneity-stabilized equilibria.

# gst: hidden qu-classical correspondence in chaotic billiards revealed by mutual information.

<< ️Avoided level crossings, commonly associated with quantum chaos, are typically interpreted as signatures of eigenstate hybridization and spatial delocalization, often viewed as ergodic spreading. >>

<< ️(AA) show that, contrary to this expectation, increasing chaos in quantum billiards enhances mutual information between conjugate phase space variables, revealing nontrivial correlations. Using an information-theoretic decomposition of eigenstate entropy, (They) demonstrate that spatial delocalization may coincide with increased mutual information between position and momentum. >>

<< These correlations track classical invariant structures in phase space and persist beyond the semiclassical regime, suggesting a robust information-theoretic manifestation of quantum-classical correspondence. >>

Kyu-Won Park, Soojoon Lee, Kabgyun Jeong. Hidden quantum-classical correspondence in chaotic billiards revealed by mutual information. Phys. Rev. E 112, 024209. Aug 13, 2025.

https://journals.aps.org/pre/abstract/10.1103/qpxx-csdj

arXiv:2505.08205v1 [nlin.CD]. May 13, 2025.

https://arxiv.org/abs/2505.08205

Also: billiard, chaos, waves, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, billiard, chaos, qu-chaos, waves, quantum-to-classical transition

# gst: transition to chaos with conical billiards.

<< ️(AA) adapt ideas from geometrical optics and classical billiard dynamics to consider particle trajectories with constant velocity on a cone with specular reflections off an elliptical boundary formed by the intersection with a tilted plane, with tilt angle γ. >>

<< ️(They) explore the dynamics as a function of γ and the cone deficit angle χ that controls the sharpness of the apex, where a point source of positive Gaussian curvature is concentrated. >>

<< ️(AA) find regions of the (γ,χ) plane where, depending on the initial conditions, either (A) the trajectories sample the entire cone base and avoid the apex region; (B) sample only a portion of the base region while again avoiding the apex; or (C) sample the entire cone surface much more uniformly, suggestive of ergodicity. >>

<< ️The special case of an untilted cone displays only type A trajectories which form a ring caustic at the distance of closest approach to the apex. However, (They) observe an intricate transition to chaotic dynamics dominated by Type (C) trajectories for sufficiently large χ and γ. A Poincaré map that summarizes trajectories decomposed into the geodesic segments interrupted by specular reflections provides a powerful method for visualizing the transition to chaos. (AA) then analyze the similarities and differences of the path to chaos for conical billiards with other area-preserving conservative maps. >>

Lara Braverman, David R. Nelson. Transition to chaos with conical billiards. arXiv: 2508.02786v1 [nlin.CD]. Aug 4, 2025.

https://arxiv.org/abs/2508.02786

Also: billiard, particle, transition, chaos,  in https://www.inkgmr.net/kwrds.html 

Keywords: gst, billiard, particles, transitions, chaos. 

# gst: an analysis of the phenomenon of chaotic itinerancy.

AA << introduce a new methodology for the analysis of the phenomenon of chaotic itinerancy in a dynamical system using the notion of entropy and a clustering algorithm. (They) determine systems likely to experience chaotic itinerancy by means of local Shannon entropy and local permutation entropy. In such systems, we find quasi-stable states (attractor ruins) and chaotic transition states using a density-based clustering algorithm. >>

Their << ️approach then focuses on examining the chaotic itinerancy dynamics through the characterization of residence times within these states and chaotic transitions between them with the help of some statistical tests. The effectiveness of these methods is demonstrated on two systems that serve as well-known models exhibiting chaotic itinerancy: globally coupled logistic maps (GCM) and mutually coupled Gaussian maps. >>

<< ️Although the phenomenon of chaotic itinerancy is often associated with high dimensional systems, (They) were able to provide evidence for the presence of this phenomenon in the studied low-dimensional systems. >>

Nikodem Mierski, Paweł Pilarczyk. Analysis of the Chaotic Itinerancy Phenomenon using Entropy and Clustering. arXiv: 2507.22643v1 [nlin.CD]. Jul 30, 2025. 

https://arxiv.org/abs/2507.22643

Also: chaos, intermittency, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, chaos, intermittency, transitions, chaotic itinerancy, low-dimensional systems.

# brain: ubiquity of uncertainty in neuron systems.

AA << demonstrate that final-state uncertainty is ubiquitous in multistable systems of coupled neuronal maps, meaning that predicting whether one such system will eventually be chaotic or nonchaotic is often nearly impossible. (They) propose a "chance synchronization" mechanism that governs the emergence of unpredictability in neuron systems and support it by using basin classification, uncertainty exponent, and basin entropy techniques to analyze five simple discrete-time systems, each consisting of a different neuron model. >>

Their << ️results illustrate that uncertainty in neuron systems is not just a product of noise or high-dimensional complexity; it is also a fundamental property of low-dimensional, deterministic models, which has profound implications for understanding brain function, modeling cognition, and interpreting unpredictability in general multistable systems. >>

Brandon B. Le, Bennett Lamb, et al. Ubiquity of Uncertainty in Neuron Systems. arXiv: 2507.15702v1 [q-bio.NC]. Jul 21, 2025.

https://arxiv.org/abs/2507.15702

Also: brain, uncertainty, noise, in https://www.inkgmr.net/kwrds.html 

Keywords: brain, uncertainty, noise, coupled neuronal maps, chance synchronization.

# gst: dynamics and chaotic properties of the fully disordered Kuramoto model.

<< ️Frustrated random interactions are a key ingredient of spin glasses. From this perspective, (AA) study the dynamics of the Kuramoto model with quenched random couplings: the simplest oscillator ensemble with fully disordered interactions. (AA) answer some open questions by means of extensive numerical simulations and a perturbative calculation (the cavity method). (They) show frequency entrainment is not realized in the thermodynamic limit and that chaotic dynamics are pervasive in parameter space.  >>

<< ️In the weak coupling regime, (AA) find closed formulas for the frequency shift and the dissipativeness of the model. Interestingly, the largest Lyapunov exponent is found to exhibit the same asymptotic dependence on the coupling constant irrespective of the coupling asymmetry, within the numerical accuracy. >>

Iván León, Diego Pazó. Dynamics and chaotic properties of the fully disordered Kuramoto model. arXiv:2507.05168v1 [cond-mat.dis-nn]. Jul 7, 2025.

https://arxiv.org/abs/2507.05168

Also: disorder & fluctuations, weak, chaos, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, disorder & fluctuations, weakness, chaos, transitions, volcano transition, slow relaxation, quasientrainment, freezing, nonreciprocity.

# gst: a note on spinning billiards and chaos

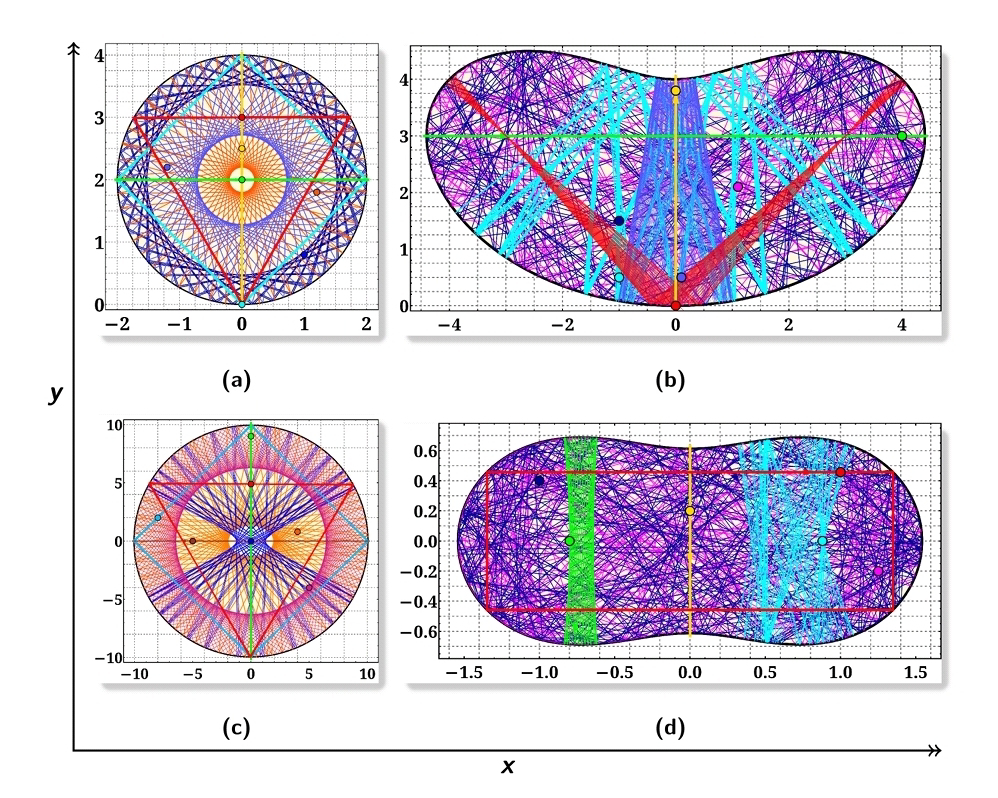
AA << investigate the impact of internal degrees of freedom - specifically spin - on the classical dynamics of billiard systems. While traditional studies model billiards as point particles undergoing specular reflection, (AA) extend the paradigm by incorporating finite-size effects and angular momentum, introducing a dimensionless spin parameter that characterizes the moment of inertia. Using numerical simulations across circular, rectangular, stadium, and Sinai geometries, (AA) analyze the resulting trajectories and quantify chaos via the leading Lyapunov exponent. >>

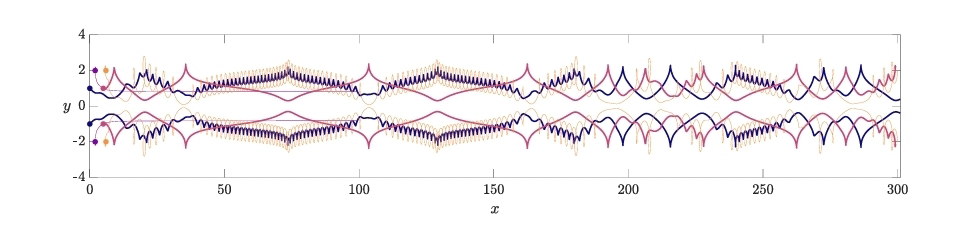
<< Strikingly, (They) find that spin regularizes the dynamics even in geometries that are classically chaotic: for a wide range of α, the Lyapunov exponent vanishes at late times in the stadium and Sinai tables, signaling suppression of chaos. This effect is corroborated by phase space analysis showing non-exponential divergence of nearby trajectories. >>

AA << results suggest that internal structure can qualitatively alter the dynamical landscape of a system, potentially serving as a mechanism for chaos suppression in broader contexts. >>

Jacob S. Lund, Jeff Murugan, Jonathan P. Shock. A Note on Spinning Billiards and Chaos. arXiv: 2505.15335v1 [nlin.CD]. May 21, 2025.

https://arxiv.org/abs/2505.15335

Also: billiard, chaos, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, billiard, spinning billiards, chaos.

# gst: unstable fixed points in chaotic networks

<< Understanding the high-dimensional chaotic dynamics occurring in complex biological systems such as recurrent neural networks or ecosystems remains a conceptual challenge. For low-dimensional dynamics, fixed points provide the geometric scaffold of the dynamics. However, in high-dimensional systems, even the location of fixed points is unknown. >>

Here, AA << analytically determine the number and distribution of fixed points for a canonical model of a recurrent neural network that exhibits high-dimensional chaos. This distribution reveals that fixed points and dynamics are confined to separate shells in state space. Furthermore, the distribution enables (AA) to determine the eigenvalue spectra of the Jacobian at the fixed points, showing that each fixed point has a low-dimensional unstable manifold. >>

<< Despite the radial separation of fixed points and dynamics, (They)  find that the principal components of fixed points and dynamics align and that nearby fixed points act as partially attracting landmarks for the dynamics. >>

AA results << provide a detailed characterization of the fixed point geometry and its interplay with the dynamics, thereby paving the way towards a geometric understanding of high-dimensional chaos through their skeleton of unstable fixed points. >>

Jakob Stubenrauch, Christian Keup, et al. Fixed point geometry in chaotic neural networks. Phys. Rev. Research 7, 023203. May 29, 2025.

https://journals.aps.org/prresearch/abstract/10.1103/PhysRevResearch.7.023203

Also: chaos, disorder & fluctuations, instability, transition, network, brain, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, chaos, networks, neural networks, ecosystems, fixed points, unstable fixed points.

# gst: elasticity of fibers prefers the chaos of turbulence.

<< The dynamics of fibers, modeled as a sequence of inertial beads linked via elastic springs, in turbulent flows is dictated by a nontrivial interplay of inertia and elasticity. Such elastic, inertial fibers preferentially sample a three-dimensional turbulent flow in a manner that is qualitatively similar to that in two dimensions [R. Singh et al., Phys. Rev. E 101, 053105 (2020)]. >>

<< Both these intrinsic features have competing effects on fiber dynamics: Inertia drives fibers away from vortices while elasticity tends to trap them inside. However, these effects swap roles at very large values. A large inertia makes the fibers sample the flow more uniformly while a very large elasticity facilitates the sampling of straining regions. >>

<< This complex sampling behavior is further corroborated by quantifying the chaotic nature of sampled flow regions. This is achieved by evaluating the maximal Lagrangian Lyapunov Exponents associated with the flow along fiber trajectories. >>

Rahul K. Singh. Elasticity of fibers prefers the chaos of turbulence. Phys. Rev. E 111, L053101. May 5, 2025.

https://journals.aps.org/pre/abstract/10.1103/PhysRevE.111.L053101

Also: elastic, turbulence, chaos, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, elasticity, turbulence, chaos, transitions

# gst: hyperchaos and complex dynamical regimes in N-d neuron lattices.

AA << study the dynamics of N-dimensional lattices of nonchaotic Rulkov neurons coupled with a flow of electrical current. (They) consider both nearest-neighbor and next-nearest-neighbor couplings, homogeneous and heterogeneous neurons, and small and large lattices over a wide range of electrical coupling strengths. >>

<< As the coupling strength is varied, the neurons exhibit a number of complex dynamical regimes, including unsynchronized chaotic spiking, local quasi-bursting, synchronized chaotic bursting, and synchronized hyperchaos. >>

<< For lattices in higher spatial dimensions, (AA) discover dynamical effects arising from the ``destructive interference'' of many connected neurons and miniature ``phase transitions'' from coordinated spiking threshold crossings. In large two- and three-dimensional neuron lattices, (They) observe emergent dynamics such as local synchronization, quasi-synchronization, and lag synchronization. >>

<< These results illustrate the rich dynamics that emerge from coupled neurons in multiple spatial dimensions, highlighting how dimensionality, connectivity, and heterogeneity critically shape the collective behavior of neuronal systems. >>

Brandon B. Le, Dima Watkins. Hyperchaos and complex dynamical regimes in N-dimensional neuron lattices. arXiv: 2505.03051v1 [nlin.CD]. May 5, 2025.

https://arxiv.org/abs/2505.03051

Also: brain, network, behavior, chaos, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, brain, network, behavior, cooperation, cooperative behavior, chaos, hyperchaos, transitions, phase transitions, transition thresholds,  synchrony, dimensionality, topology of connectivity, intermittent bursting activity, interference, destructive interference.

# gst: period-doubling route to chaos in viscoelastic flows

<< Polymer solutions can develop chaotic flows, even at low inertia. This purely elastic turbulence is well studied, but little is known about the transition to chaos. In two-dimensional (2D) channel flow and parallel shear flow, traveling wave solutions involving coherent structures are present for sufficiently large fluid elasticity. >>

AA << numerically study 2D periodic parallel shear flow in viscoelastic fluids, and (They) show that these traveling waves become oscillatory and undergo a series of period-doubling bifurcations en-route to chaos. >>

Jeffrey Nichols, Robert D. Guy, Becca Thomases. Period-doubling route to chaos in viscoelastic Kolmogorov flow. Phys. Rev. Fluids 10, L041301. Apr 17, 2025.

https://journals.aps.org/prfluids/abstract/10.1103/PhysRevFluids.10.L041301

Also: chaos, waves, elastic, turbulence, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, chaos, waves, traveling waves, elasticity, viscoelastic fluids, turbulence, elastic turbulence, period-doubling bifurcations, transitions

# gst: stochastic surfing turbulent vorticity.

<< The chaotic dynamics of small-scale vorticity plays a key role in understanding and controlling turbulence, with direct implications for energy transfer, mixing, and coherent structure evolution. >>

Here AA << use a combination of experiments, theory and simulations to show that small magnetic particles of different densities, exploring flow regions of distinct vorticity statistics, can act as effective probes for measuring and forcing turbulence at its smallest scale. The interplay between the magnetic torque, from an externally controllable magnetic field, and hydrodynamic stresses, from small-scale turbulent vorticity, reveals an extremely rich phenomenology. >>

Notably, AA << present the first observation of stochastic resonance for particles in turbulence: turbulent fluctuations, effectively acting as noise, counterintuitively enhance the particle rotational response to external forcing. (They) identify a pronounced resonant peak in particle rotational phase-lag when the applied magnetic field matches the characteristic intensity of small-scale vortices. >>

<< Furthermore, (They) uncover a novel symmetry-breaking mechanism: an oscillating magnetic field with zero-mean angular velocity remarkably induces net particle rotation in turbulence with zero-mean vorticity, as turbulent fluctuations aid the particle in "surfing" the magnetic field. >>

Ziqi Wang, Xander M. de Wit, et al. Stochastic surfing turbulent vorticity. arXiv: 2504.08346v1 [physics.flu-dyn]. Apr 11, 2025. 

https://arxiv.org/abs/2504.08346

Also: vortex, turbulence, noise, transition, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, vortices, turbulence, turbulent fluctuations, small-scale turbulent vorticity, stochastic resonance, noise, transitions 

# gst: strange attractors in complex networks

<< Disorder and noise in physical systems often disrupt spatial and temporal regularity, yet chaotic systems reveal how order can emerge from unpredictable behavior. Complex networks, spatial analogs of chaos, exhibit disordered, non-Euclidean architectures with hidden symmetries, hinting at spontaneous order. Finding low-dimensional embeddings that reveal network patterns and link them to dimensionality that governs universal behavior remains a fundamental open challenge, as it needs to bridge the gap between microscopic disorder and macroscopic regularities. >>

<< Here, the minimal space revealing key network properties is introduced, showing that non-integer dimensions produce chaotic-like attractors. >>

Pablo Villegas. Strange attractors in complex networks. Phys. Rev. E 111, L042301. Apr 15, 2025. 

https://journals.aps.org/pre/abstract/10.1103/PhysRevE.111.L042301.  

arXiv: 2504.08629v1 [cond-mat.stat-mech] . Apr 11, 2025.

https://arxiv.org/abs/2504.08629

Also: disorder, disorder & fluctuations, noise, network, attractor, chaos, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, disorder, disorder & fluctuations, noise, networks, attractors, self-similarity, chaos 

# gst: weird quasiperiodic attractors

AA << consider a class of n-dimensional, n≥2, piecewise linear discontinuous maps that can exhibit a new type of attractor, called a weird quasiperiodic attractor. While the dynamics associated with these attractors may appear chaotic, (They)  prove that chaos cannot occur. The considered class of n-dimensional maps allows for any finite number of partitions, separated by various types of discontinuity sets. The key characteristic, beyond discontinuity, is that all functions defining the map have the same real fixed point. These maps cannot have hyperbolic cycles other than the fixed point itself. >>

Laura Gardini, Davide Radi, et al. Abundance of weird quasiperiodic attractors in piecewise linear discontinuous maps. arXiv: 2504.04778v1 [math.DS]. Apr 7, 2025.

https://arxiv.org/abs/2504.04778

Also: attractor, chaos, in https://www.inkgmr.net/kwrds.html 

Keywords: gst, attractors, weird attractors, chaos